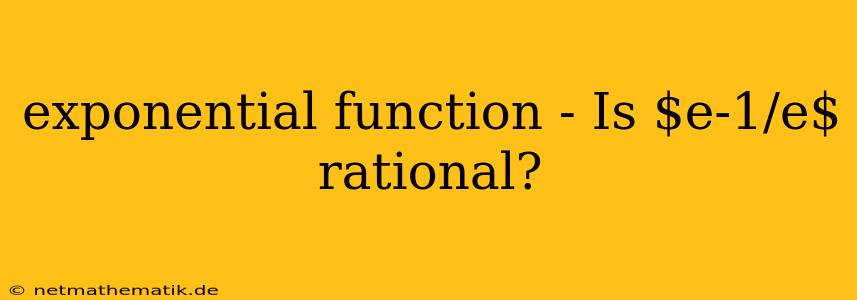
The exponential function, denoted by $e^x$, is one of the most fundamental functions in mathematics. It appears in various areas of science, engineering, and finance. A key property of the exponential function is that its derivative is itself, making it essential for modeling continuous growth or decay. In this context, we will investigate the nature of the expression $e-1/e$. Specifically, we will address the question: Is $e-1/e$ rational?
Understanding Rational Numbers
Before diving into the analysis of $e-1/e$, it's crucial to understand the concept of rational numbers. A rational number is a number that can be expressed as a ratio of two integers, where the denominator is not zero. For example, 3/4, -2/5, and 7 are all rational numbers. Conversely, a number that cannot be expressed in this form is considered irrational. Famous examples of irrational numbers include $\pi$ and $\sqrt{2}$.
Exploring the Properties of 'e'
The number 'e' is a mathematical constant approximately equal to 2.71828. It is often referred to as Euler's number, named after the Swiss mathematician Leonhard Euler. 'e' is an irrational number, meaning it cannot be expressed as a simple fraction of two integers. This fact plays a critical role in determining the rationality of $e-1/e$.
Analyzing the Expression $e-1/e$
To determine whether $e-1/e$ is rational, we can attempt to express it as a fraction of two integers. Let's assume, for the sake of contradiction, that $e-1/e$ is rational. This means we can write it as:
$e - \frac{1}{e} = \frac{p}{q}$
where $p$ and $q$ are integers, and $q$ is not zero. Multiplying both sides of the equation by $e$ and rearranging terms, we obtain:
$e^2 - \frac{p}{q}e - 1 = 0$
This is a quadratic equation in terms of 'e'. We can use the quadratic formula to solve for 'e':
$e = \frac{\frac{p}{q} \pm \sqrt{(\frac{p}{q})^2 + 4}}{2}$
Now, we arrive at a critical point. Since 'e' is irrational, it cannot be expressed as a fraction of two integers. However, the quadratic formula above implies that 'e' can be expressed as a fraction involving $p$, $q$, and the square root of a rational number. This contradiction arises from our initial assumption that $e-1/e$ is rational.
Conclusion: $e-1/e$ is Irrational
Based on the analysis above, we conclude that $e-1/e$ is irrational. Our initial assumption that it could be expressed as a fraction of two integers led to a contradiction with the known irrationality of 'e'. This demonstrates that the expression $e-1/e$ cannot be simplified to a rational form.
The exploration of the rationality of $e-1/e$ provides a valuable illustration of how the properties of irrational numbers can influence the nature of mathematical expressions involving them. Understanding the difference between rational and irrational numbers is fundamental to various mathematical concepts, including calculus, analysis, and number theory.